Table of Contents
Gravity waves
Author: Leonardo Echeverria
These are called gravity waves because gravity is the restoring force that perpetuates them [2]. Examples of these are surface waves, which are the result of the interaction between wind and the ocean; and tides, which are the result of gravitational attraction of the sun and the moon [4].
General Definitions
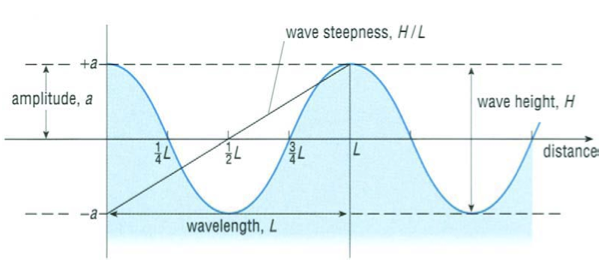
$c$ : celerity of propagation, wave speed, or phase speed is the speed of an individual wave
$$c = \sqrt{\frac{g L}{2\pi}tanh(\frac{2\pi d}{L})} = \frac{L}{T}$$
$L$ : Wave length
$T$ : Wave period
$f=1/T$ : Frequency
$k=\frac{2\pi}{L}$ : Wave number
$\sigma = \frac{2\pi}{T}$ : Angular frequency
The rate of the advance of wave energy is known as Group Velocity ($c_{g}$) [2]
$c_{g}= \frac{\sigma_{1}-\sigma_{2}}{k_{1}-k_{2}}$
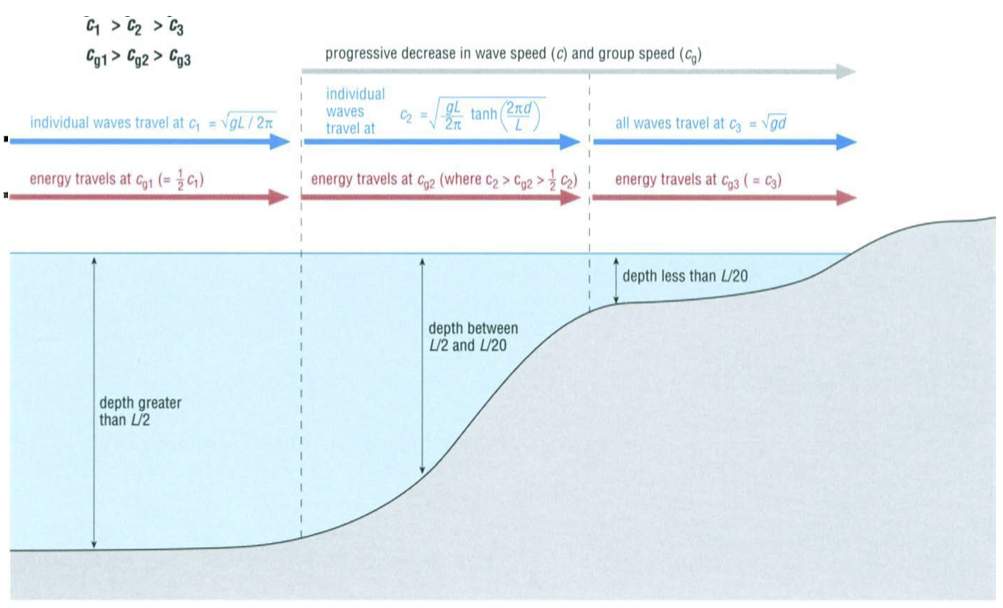
Deep Water Waves
A wave is considered to be a deep water wave as long as water depth ($d$) exceeds 1/2 of wavelength ($L$) [3]
Individual wave speed is 2 times group velocity [3].
The diameters of particle orbits decrease exponentially downwards[4].
Shallow Water Waves
A wave is considered to be a shallow water wave as long as water depth ($d$) is less than 1/20 of wavelength ($L$) [3]
Group velocity for shallow water waves equals individual wave speed [3].
The horizontal diameters remain constant but ellipticity increases downwards [4].
From Deep Water to Shallow Water Waves
As a wave approaches the shore shallow water waves are developed.
We can distinguish three zones:
Deep water zone ($d>L/2$),
Transition zone ($L/20<d <L/2$), and
Shallow water zone ($d<L/20$)
 [2]
[2]
Refraction
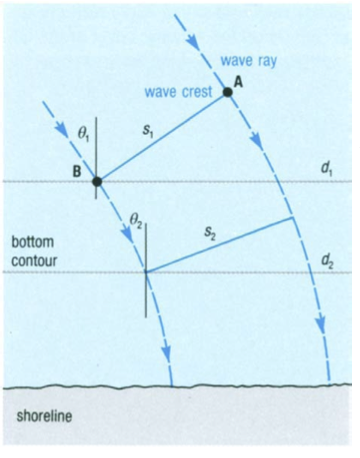 Refraction is the bending of wave energy due to bottom friction.
Refraction is the bending of wave energy due to bottom friction.
In areas with sharp variations in coastline, refraction focuses the wave energy at the tips of points and headlands and spreads wave energy in bays and inlets.
Snell's Law
$\frac{sin(\theta_{1})}{sin(\theta_{2})}=\frac{c_{1}}{c_{2}}=\sqrt{\frac{d_{1}}{d_{2}}}$
Wave energy must be equal
$E_{1}s_{1}=E_{2}s_{2}$
Since energy is proportional to the square of height
$H_{1}^2s_{1}=H_{2}^2s_{2}$
G1FIBuybN78
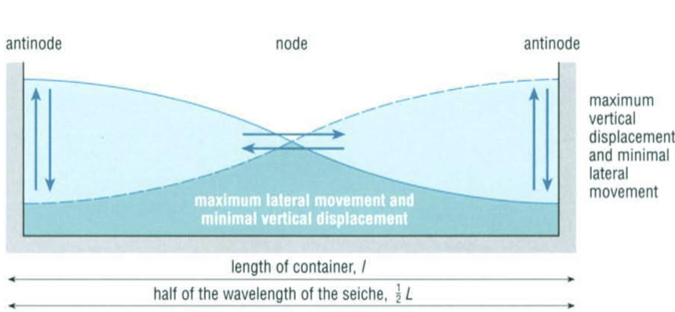
Seiches
A seiche is a standing wave that oscillates back and forth within a water body. These can occur in lakes, bays, estuaries, and harbors.
The period is determined by the length and depth
$T = \frac{1}{2n+1}\frac{2l}{\sqrt{gH}}, n =0,1,2...$
Suggested topics:
- What are gravity waves and what is their phase velocity (general)? How to distinguish shallow water and deep water waves?
- What is a shallow water wave? What assumptions were made? What is its phase velocity? Examples for shallow water waves.
- What is a deep water wave? What assumptions were made? What is its phase velocity? Examples for deep water waves.
Author: Leonardo Echeverria
References
[2] University Corporation for Atmospheric Research Wave Types and Characteristics
[3] University Corporation for Atmospheric Research Shallow Water Waves
[4] Open University,Chapter 1: Waves, Tides and Shallow-Water Process